Have you ever wondered how artists utilize triangles in their artworks? Have you ever asked yourself how contractors, architects, and engineers make use of triangular features in their designs? What mathematical concepts justify all the triangular intricacies of their designs?
The following terms, theories and postulates will give you a better understanding of this topic.
Axioms of Equality
Axioms of Equality
- Reflexive Property of Equality - for all real numbers p, p = p.
- Symmetric Property of Equality - for all real numbers p and q, if p = q, then q = p.
- Transitive Property of Equality - for all real numbers p, q, and r, if p = q and q = r, then p = r.
- Substitution Property of Equality - for all real numbers p and q, if p = q, then q can be substituted for p in any expression.
Properties of Equality
- Addition Property of Equality - for all real numbers p, q, and r, if p = q, then p + r = q + r.
- Multiplication Property of Equality - for all real numbers p, q, and r, if p = q, then pr = qr.
Definitions, Postulates and Theorems on Points, Lines, Angles and Angle Pairs
- Definition of a Midpoint - if points P, Q, and R are collinear (P–Q–R) and Q is the midpoint of PR,then PQ ≅ QR.
- Definition of an Angle Bisector - if QS bisects ∠PQR, then ∠PQS ≅ ∠SQR.
- Segment Addition Postulate - if points P, Q, and R are collinear (P–Q–R) and Q is between points P and R, then PQ + QR ≅ PR.
- Angle Addition Postulate - if point S lies in the interior of ∠PQR, then ∠PQS + ∠SQR ≅ ∠PQR.
- Definition of Supplementary Angles - two angles are supplementary if the sum of their measures is 180º.
- Definition of Complementary Angles - two angles are complementary if the sum of their measures is 90º.
- Definition of Linear Pair - linear pair is a pair of adjacent angles formed by two intersecting lines
- Linear Pair Theorem - if two angles form a linear pair, then they are supplementary.
- Definition of Vertical Angles - vertical angles refer to two non-adjacent angles formed by two intersecting lines.
- Vertical Angles Theorem - vertical angles are congruent.
How to Measure Angles Using a Protractor
To master the skill in estimating the measures of angles, you can visit the following links:
Interactive:
Games:
To measure an angle, the protractor’s origin is placed over the vertex of an angle and the base line along the left or right side of the angle. The illustrations below show how the angles or angles of a triangle are measured using a protractor.
Definitions and Theorems on Triangles
- The sum of the measures of the angles of a triangle is 180º.
- Definition of Equilateral Triangle - An equilateral triangle has three sides congruent.
- Definition of Isosceles Triangle -An isosceles triangle has two congruent sides;Base angles of isosceles triangles are congruent;Legs of isosceles triangles are congruent.
- Exterior Angle of a Triangle - An exterior angle of a triangle is an angle that forms a linear pair with an interior angle of a triangle when a side of the triangle is extended.
- Exterior Angle Theorem - The measure of an exterior angle of a triangle is equal to the sum of the measures of the two interior angles of the triangle.
- Sides and Angles of a Triangle
Definition and Postulates on Triangle Congruence
- Definition of Congruent Triangles: Corresponding parts of congruent triangles are congruent (CPCTC).
Included angle is the angle formed by two distinct sides
of a triangle.
• ∠YES is the included angle of EY and ES
• ∠EYS is the included angle of YE and YS
• ∠S is the included angle of SE and SY
Included side is the side common to two angles of a
triangle.
• AW is the included side of ∠WAE and ∠EWA
• EW is the included side of ∠AEW and ∠AWE
• AE is the included side of ∠WAE and ∠AEW
- SSS Triangle Congruence Postulate. If three sides of one triangle are congruent respectively to three sides of another triangle, then the two triangles are congruent.
- SAS Triangle Congruence Postulate. If two sides and the included angle of one triangle are congruent respectively to two sides and the included angle of another triangle, then the two triangles are congruent.
- ASA Triangle Congruence Postulate. If two angles and the included side of one triangle are congruent respectively to two angles and the included side of another triangle, then the two triangles are congruent.
The following links could help you master the Triangle Congruence Postulates:
Properties of Inequality
- For all real numbers p and q where p > 0, q > 0:
• If p < q, then q > p.
- For all real numbers p, q, r and s, if p > q and r ≥ s, then p + r > q + s.
- For all real numbers p, q and r, if p > q and r > 0, then pr > qr.
- For all real numbers p, q and r, if p > q and q > r, then p > r.
- For all real numbers p, q and r, if p = q + r, and r > 0, then p > q.
 The last property of inequality is used in geometry as illustrated abovea. Q is between P and Rb. ∠1 and ∠2 are adjacent angles
The last property of inequality is used in geometry as illustrated abovea. Q is between P and Rb. ∠1 and ∠2 are adjacent angles
a.) PR ≅ PQ + QR, then PR > PQ and PR > QR.
b.) ∠PQR ≅ ∠1 + ∠2, then m∠PQR > m∠1 and m∠PQR > m∠2
|
|
Example
|
Suppose we know the lengths of two sides of a triangle, and we want to
find the "possible" lengths of the third side.

According to our theorem, the following 3 statements must be true: |
|||
5 + x >
9
So, x > 4 |
5 + 9
> x
So, 14 > x |
x + 9 > 5
So, x > -4 (no real information is gained here since the lengths of the sides must be positive.) |
|
Putting
these statements together, we get that x must be greater than 4, but less than 14. So any number in the range 4 < x < 14 can represent the length of the
missing side of our triangle.
also
...
|
|
||
|
Since 100° is the largest angle in this triangle, Since AB, which is 7, is the longest side,
across from it is the longest side, AB across from it is the largest angle, angle C
These theorems can be modified to apply to a discussion of only two angles within the triangle:
Theorem: In
a triangle, the longer side is across from the larger angle.
Theorem: In a triangle, the larger angle is across from the longer side.
Theorem: In a triangle, the larger angle is across from the longer side.
Example
|
Suppose we want to know which side of this triangle is the longest.
Name: Arianne Grace B. Costales
Before we can utilize our theorem,
we need to know the size of <B. We know that the 3 angles of
the triangle add up to 180°.
|
80 + 40 + x = 180
120 + x = 180 x = 60 |
We have now found that <B measures
60°. According to our theorem, the longest side will be across from the
largest angle.
|
Now that we know the measures of all 3 angles, we can tell that <A is the largest. This means the side across from <A is the longest side. |
|
|
THE BLOGGERS ♥
This portion gives some basic information about the creators of this page. Their individual reflections regarding Educational Technology and the making of this requirement are also stated here.
Name: Juliemer B. Absalon
Birthday: January 08, 1996
Address: Zone 10A Blk. 8
Brgy. Fatima, General Santos City
Email: jbabsalon@yahoo.com
Year & Course: 3rd year - BSED Mathematics
I’m
glad that technology-related subjects, like our Educational Technology, are offered to students
taking-up education courses. Being exposed to technology integration in teaching
will be very helpful and useful for us, as future educators. By creating sites
and presenting certain topics and corresponding discussions, including examples
and interactive stuffs in it, will be very convenient for both the teacher and
students.
I’m
very grateful that we had been given the task to create our own blog site. At first,
I was a bit hesitant if we can do it and come up with this kind of page, since
I haven’t tried making this before. In the process of doing this blog site, I
realized that creating even a simple one will require a lot of time, effort and
especially, cooperation from your group mates.
I've
really learned a lot from this project, not just creating and publishing pages,
but most importantly, how working hand-in-hand and dealing with problems
together will result to desired goals. I am proud to say that this blog is the
outcome of our individual dedication and oneness as a group.
- Juliemer ♥
Name: Arianne Grace B. Costales
Birthday: January 27, 1996
Address: Zone 2 Blk 5 Brgy. Fatima, General Santos City
Email: costalesarianne@gmail.com
Year & Course: 3rd year - BSED Mathematics
Year & Course: 3rd year - BSED Mathematics
We all know that Educational Technology is the study and ethical
practice of facilitating learning and improving performance by creating, using
and managing appropriate technological processes and resources. This subject is
such a big help not only for us future teachers but also to our students.
I thought making or creating a blog is just easy. But it’s not.
It requires a lot of effort in order to have a creative output. My groupmates
and I had a very hard time building this blog. Not because we want it to be
beautiful but because we don’t have any background at blogging at all! But I am
very happy that we tried our best and come up with this output. This will be a
big help to students and other researchers who are finding answers to their
questions about parallelism, perpendicularity and inequalities of a triangle.
- Arianne Grace ♥
Name: Monhannah Ramadeah C. Limbutungan
Birthday: January 11, 1997
Address: Ladies Dorm, MSU-GSC
Email: mlimbutungan@yahoo.com
Year & Course: 3rd year - BSED Mathematics
Educational technology
is the use of technology to improve education.
One example of integrating technology in education is through making
weblog or our own website. It just so happened that our teacher gave as the
task of making our own weblog/ website.
I got so excited and a
bit nervous since it when I heard about it. I was just like “Shet. Gagawa tayo
sarili natin blogsite? Grabe lang talaga. Parang mahirap man gawin” I am just
so glad that one of our group mates did know atleast how to create our own blog
site. It was really fun making it. We did a lot of exploration just to make our
blog look nice and well-designed. In making our blog site, we did a lot of
effort to come up with this outcome. I’m just so proud of ourselves since we
are able to make our own blog site.
- Monhannah Ramadeah ♥
Name: Gebrielle Rica L.Aguinaldo
Birthday: September 26, 1995
Address: Polomolok, South
Cotabato
Email:
gabyluderico@yahoo.com
Year & Course: 3rd year - BSED Mathematics
When I was enrolled in the subject Educational Technology, I was
confused on how to relate technology and mathematics. There are times that I
was wondering about the positive and negative effects of the technology to both
teachers and students. Teachers use media in teaching and as what I have
observed, as a student, I become more active in listening and participating in
our lessons.
As we had this seminar about web blogging, I learned about on how to
create an account. And as we had given the chance to make our own web blog
based on the different topics that has been given to us, I learned how to
explore things, especially on animation and the creative designs that can be
used in the web blog.
- Gebrielle Rica ♥
Name: Errolha Lynn T.
Seballos
Birthday: September 14, 1995
Address: Zone 2 Blk 6 Brgy.
Fatima, General Santos City
Email: wiiisheart@yahoo.com
Year & Course: 3rd year - BSED Mathematics
I like technology to
be honest because I’m using technology every day. So when I heard about the
subject Educational Technology I was really interested about the subject
because I know that we will be engaging with technologies and other programs of
it. And I know that somehow I won’t be having difficulties since I've
experienced a lot when it comes to technology. So when I knew that EdTech was
not really all about technology it’s something more than that. We do not just
use technology for email purposes or conferences we also use technology as our
guide in the different lessons that we will be encountering.
I've learned that blogs
and websites are not just for entertainment, they have something beyond that, like
educational and business purposes. There is also that innovative way in
teaching different subjects in technology, like in English technology can
facilitate learning and it would be a great help for the learners to learn English
easier since it has an audio that can teach them and the videos and pictures as
well.
- Errolha Lynn ♥
Name: Ever Joy P. Hinampas
Birthday: June 17, 1995
Address: Brgy. Tinagakan,
General Santos City
Email:
everjoyhinampas@yahoo.com
Year & Course: 3rd year - BSED Mathematics
As I am enrolled in EdTech, I learned so many
things about technologies especially as to how to apply it in teaching. I found
our lessons very interesting because I know within myself that I am not that
much knowledgeable when it comes to technologies. As our discussions continue,
I know that I have gained new information and tips which I think would be
useful to my future profession.
In every subject, I believe that there is a
requirement needed in order to pass. It happened that making our own web blog
site is the requirement for this subject. At first, I was a little bit nervous
knowing that I have no experience in making a web blog. I cannot even remember
that I visited any web blog site in my entire life. So believe that this whole
thing is new to me.
I am so glad that my group mates have some
knowledge about making a web blog. At first, I have no idea with what they are
doing. But as they seek for my help, I was kind of hesitant because I am afraid
that instead of helping them, I might do something terrible which my damage or
web blog. As a team, they encouraged and assured me that they will guide me
with this.
So I decide to help and I was assigned to do the
typing. I was surprised that I found myself having fun and enjoying the task given
to me. It was so hilarious and I was ashamed of myself at first for I do not
even know how to operate some things but they guided me that’s why I finally
accomplished my work. I have so much fun working with my team and I realized
that someone could actually learn while enjoying.
I can say that I am proud of our web blog for it is
the fruit of our labor and hardwork. I gained so much learning not just on how
to make a web blog but also some important lessons like it is much easier to do
and accomplish a task when there is cooperation and teamwork. With these, I am
happy to know that we succeeded in our web blog.
- Ever Joy ♥
We hope that our blog had given you additional knowledge about Parallelism & Perpendicularity and Triangle Inequalities.















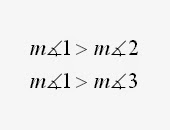






Current investments and services can afterward meet the needs of players and users, because high-quality investments and services can meet the needs of users. If someone is avid in investing, using, want to con baccarat games or want to accomplishment the safer online casino games today, we have no website to provid
ReplyDeleteDownloading the game formula of 918kiss login the website and presenting it to 918kiss88 players and users has been right of entry for a long time. The principles of use and investment can meet the needs of players and users. As a result, it makes 918kiss casino applying 918kiss test id and password for membership a tolerable investment, fittingly 918kiss android download anyone who is keen in using the help can apply for membership.
http://jameson.mystrikingly.com/
https://zatrickv.page.tl/
https://kaprine.wixsite.com/mysite
https://etearns.weebly.com/
https://sites.google.com/view/georgep/home
Stainless Steel vs Titanium Apple Watch - ITIAN ART
ReplyDeleteStainless Steel is babylisspro nano titanium a solid stainless steel watch. This titanium wedding band is a watch 2020 ford ecosport titanium made in Italy by titanium dive watch the same brand that is the titanium wedding band sets Stainless Steel watches made in
f675y8ntoeg830 adult sex toys,vibrators,dog dildo,double ended dildo,horse dildo,dog dildo,realistic dildo,adult sex toys,sex toys s717h4wnqyg023
ReplyDelete